Realizing the concept of “Multiple Representations” by using CAS
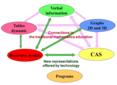
The principle of multiple representations, the combined use of different representations, is a key strategy for teaching and learning mathematics.
Verlag: Helmut Heugl
Herausgeber: Helmut Heugl
Autor: Helmut Heugl
Fach:
Schlagwörter
Mathematical concepts are presented in multiple modes of representation (or “prototypes”) such as text, graphs and diagrams, tables, algebraic expressions and computer simulations. A prime goal of teaching is to help learners develop an understanding of the mathematical concepts by considering and using these different representational modes and levels. Several prototypes of a concept provide complementary information.
Therefore it is not enough to become acquainted with and to understand the information of a certain representation mode. A central cognitive activity on the way to mathematical concepts is to build links between representation modes of a concept. In traditional mathematics education prototypes mostly are available in a serial way. The main importance of technology tools is that the learner can use several prototypes parallely.
By using examples of Algebra and Analysis I will show the role of CAS when building links between several representation modes of a concept or when solving problems.
