TI-Nspire CAS in Engineering Mathematics: Unit Step and Impulse - Spring-mass Problems
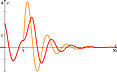
Differential equations with piecewise defined functions.
Verlag: T³ Europe
Autor: Michel Beaudin, T³ Europe
Fach: MINT
Schlagwörter Tipps und Tricks
In engineering mathematics, we often encounter a differential equation whose right hand side is a piecewise function or an impulse function. For example, a damped spring-mass oscillator. It consists of an object of mass m>0 attached to a spring fixed at one end. Applying Newton’s second law and Hooke’s law (let k>0 denote the spring constant), adding (or not) some friction proportional to velocity (let b ≥ 0 be the factor) and an external force x(t), one can obtain the following differential equation for the position y(t):
When we apply the Laplace transforms to both sides of the equation, it is transformed into the following algebraic equation where X(s)and Y(s) denote respectively the Laplace transforms of x(t) and y(t):
To be able to use the tns file below, you need to save "ETS_specfunc.tns" in the TI-Nspire CAS "Mylib" folders and refresh your libraries.
